Triangle and Its Medians
By
Cassian Mosha
Construct a triangle and its
medians. Construct a second triangle with the three sides having the lengths of
the three medians from your first triangle. Find some relationship between the
two triangles. (E.g., are they congruent? similar? have same area? same
perimeter? ratio of areas? ratio or perimeters?) Prove whatever you find.
First LetΥs construct triangle
ABC with its medians.

ΚΚΚΚΚΚΚΚΚΚΚΚΚΚFigure 1
Now we will construct another triangle using the medians. The easiest way to do it is to do it is to copy one of the median segments as a vector and translate it through one point say C
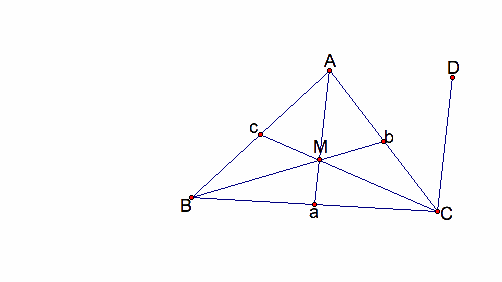
ΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚFigure 2
Now by marking point C we can translate median Aa and find another point that marks the same length as Aa. Then we will connect the point and construct a parallel line through point c that will intersect point D
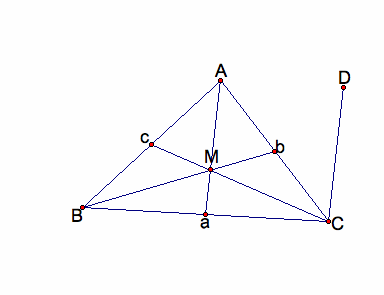
ΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚFigure 3
Now we have constructed triangle CcD which is made of same size lengths as the lengths of the medians. The figure below shows the triangle and we are ready to start exploring what we have just constructed.

ΚΚΚΚΚΚΚΚΚΚ ΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚFigure 4
The figure below shows the paralellogram BcDb that was formed, and we need to show it or prove that both sides are equal and the opposite angles are congruent.
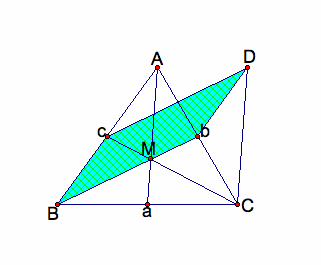
ΚΚΚΚΚΚΚFigure 5
Now let's try to prove tha the figure shaded above is a parallelogram. Suppose you are given figure BcDb, and if cD is parallel to Bb, and Bc is parallel to bD, then segment cD is equal to segment Bb and angle cDb is equal to angle and angle DcB is equal to angle DbB. To prove this let's construct a transversal line BD that divides the parallelogram into two triangles as shown on the picture below, that is triangle BcD and triangle BbD.

Figure 6
Now since cD is parallel to Bb it follows that angle cDB is equal to angle bBD because they are alternating interior angles. Same as angles bDB and cBD. It then implies that the sum of angles cDB and BDb is equal to the sum of angles cBD and bBD, thus implying that angle cDb is equal to cBb. By ASA (angle side angle theorem) triangle BcD is congruent to triangle BbD, thus side cD is congruent to side Bb, and side cB is congruent to side bB, thus figure BcDb is a parallelogram.
In terms of measurements, triangle DcB has a perimeter of 16.7cm and area of 3.92 square cm. Triangle BbD has a perimeter of 16.7cm, and an area of 3.92square cm showing that both triangles are congruent as proved above. The ratio of perimeters of the triangles is equal to 1and the ratio of their areas is 1. Again the ratio of their areas and perimeters is the same so it once again prove that the two triangles are equal or congruent. The area of the parallelogram is 7.86 square centimeters, and its perimeter is 16.94cm.
ΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚ ΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚΚ
ΚΚΚΚΚΚΚΚΚΚΚΚ